Table of Contents

• Prequiz...
• The Power Function
• 1: Introduction
• 2: What are scaling relationships?
• 3: The power function
• 4: The shape of the power function
• 5: The conversion factor "a"
• 6: The power factor "b"
• Log Transformations
• 7: Introduction
• 8: What are logarithms again?
• 9: Graphing on a log scale
• 10: Visualizing the data
• 11: Getting to a straight line
• 12: The key parameter is "b"
• The value of "b"
• 13: Introduction
• 14: A geometric approach
• 15: Scaling surface area of cubes
• 16: Scaling surface area of cubes
• 17: A "2/3 scaling law"?
• Confronting ideas with data
• 18: Introduction
• 19: An unexpected result
• 20: A "3/4 scaling law"?
• 21: Internal transport systems
• 22: Branching and supply networks
• 23: Deriving the "3/4 scaling law"
• 24: So what's the real value of "b"?
• Review
• 25: Review of Concepts

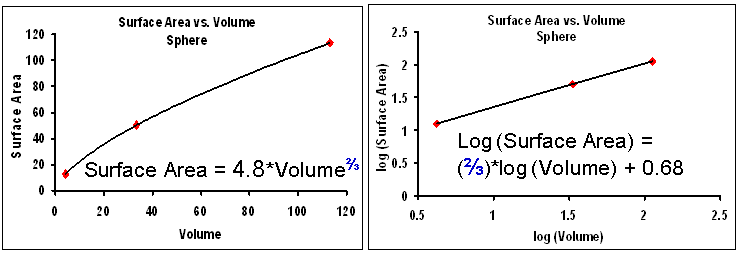
Spheres scale at 2/3 also

Wow! It turns out that when you fit the data for this relationship, the value of "a" is different, but the value of "b" has the same value for spheres and cubes: ⅔

Here again are the data for spheres using both the normal power function and the log-transformed function:!

Power function	Log-transformed function
Y = aXb	log(Y) = b*log(X) + log(a)

COOL! Surface area scales to volume to the ⅔ power!

And it turns out, this is true for any shape with a smooth surface. While the value of "a" varies from shape to shape (you can even figure out the value for each geometric shape if you want to!), the value for "b" remains constant at ⅔. This makes sense if you think about it - because surface area and volume are both calculated from a measurement of length, but surface area is always proportional to length squared while volume is always proportional to length cubed. Since we are dividing surface area (calculated from a squared quantity) by volume (calculated from a cubed quanitity) - we always end up with a ⅔ power relationship, no matter the shape.

Now we understand the mathematics behind why people thought that metabolic rate and metabolism would scale with a ⅔ power relationship. They thought it was related to how volume and surface area grew at different rates as organisms got larger. In the next section, you will see what happens when people began measuring the value of "b" - they got an unexpected result - that metabolism scaled with body size with a 3/4 power relationship. And that led to some new theories about what biological processes were limiting metabolic rates.