MathBench > Cellular Processes

Nernst Potential

 

How about those logs?

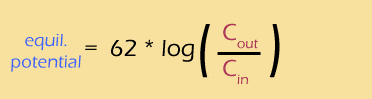
nernst eqation

I just can't leave this topic without talking at least a little about logs. Sure, when you're just plugging numbers into an equation, you can (should) ask your calculator to do the logs for you. But if you want to understand how the Nernst potential and the concentration gradients are actually related, you really should understand how that log works.

Multiplying and Adding

Last time we met logs, we were back in the scaling module. A dumb but fairly effective way to remember what logs are is to think of the log as being the length of a log that would be necessary to hold the number, like this:

log log
log size 5 (to hold 5 zeros)
log size 9 (to hold 9 zeros)

 

The important point here is that you only need a slightly bigger log to hold a much bigger number. Put another way, every time you MULTIPLY the number by 10, you only ADD 1 to the log.

So what does this mean for the Nernst potential?

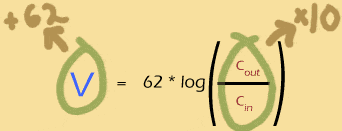
In order to increase the Nernst potential by 62mV, you must multiply the outer concentration of the ion by 10 (or, equivalently, divide the inner concentration by 10).

Take home message: Concentration gradient and Nernst potential are NOT proportional to each other! If you double the outer concentration, you do NOT double the Nernst potential.

Under ordinary conditions, ion X+ has a Nernst potential of -50mV. What will happen to the Nernst potential if the concentration of X+ outside the cell increases by 100 times?

(To make this problem interactive, turn on javascript!)

I think I have the answer: 74mV

and (don't skip this one)...

Under ordinary conditions, ion Y+ has a Nernst potential of -50mV. What will happen to the Nernst potential if the concentration of Y+ outside the cell increases by 5 times?

(To make this problem interactive, turn on javascript!)

I think I have the answer: -6.6mV

 

What goes up must come down

If multiplying the concentration gradient by ten adds 62mV to the Nernst potential, then its a good bet that dividing the gradient by 10 will subtract 62mV from the Nernst potential. Makes sense:

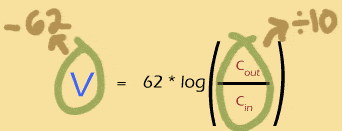
Logs of numbers less than 1

The log of 1 is 0 -- because there are no zeros or other digits after the 1. But what about the logs of measurements smaller than 1? They get a minus sign (to show that the measurement has only decimal places) but the absolute value of the log tells you how many decimal places -- so log(0.001) is -3, but log(0.000000001) is -9.

log log
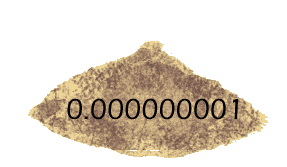log size -3 (to hold 3
decimal places)
log size -9 (to hold 9
decimal places)

 

So when will we be taking the log of a number smaller than 1?

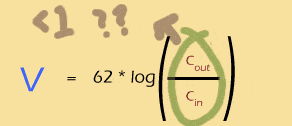
Whenever Cin is bigger than Cout, the log will be negative.

Whenever Cout is bigger than Cin, the log will be positive.

If the two are exactly equal, the log will be 0 and so will the Nernst potential.

The bigger the imbalance, the bigger the Nernst potential (either + or -).

So you can see that the log of a ratio is just about perfect for thinking about the ins and outs of a system like this.

The ion Z+ has a concentration of 125mM outside of the cell and 25mM inside. Will the Nernst potential be positive or negative (no calculator please)!

(To make this problem interactive, turn on javascript!)

I think I have the answer: positive