Table of Contents

• Prequiz...
• Part I Two Opposing Gradients
• 1: Getting through membranes
• 2: Going with the flow
• 3: Permeability is key!
• 4: Meet the neighborhood
• 5: Getting groovy with gradients
• 6: Some real numbers...
• Part II Two Useful Equations
• 7: The Goldman Equation
• 8: Playing with the Goldman Equation
• 9: The Nernst Equation
• 10: Taking Nernst for a test drive
• 11: More about Nernst
• 12: How about those logs?
• 13: Pumps and channels
• Part III The Action Potential
• 14: At equiliubrium? Or far from it?
• 15: Openning the gate
• 16: The action potential and the Goldman equation
• 17: Action potential and the Nernst equation
• Part IV Review
• 18: Summary

 

More about Nernst

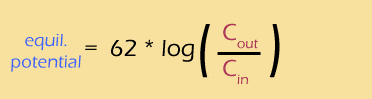
On the previous page, we found Nernst potentials for the two main cations in your cells, and they were quite different:

ion	potential
K+ Nernst potential	-90mV
Na+ Nernst potential	+72mV
real cell membrane resting potential	-60 to -80 mV

As you can see from the last line of the table, the Nernst potentials we found for the two ions don't even match the resting potential of a real cell membrane! So what gives?

Actually, what's going on is that the Nernst potential is a kind of idealisation -- even a fantasy, if you will -- that is only valid under the two conditions:

1. The membrane is permeable to the ion under consideration; and
2. The membrane is NOT permeable to any other ion

We know that membranes are not as simple as this, but in fact the resting potential of most cell membranes is somewhere close to the Nernst potential for K⁺ ... that suggests that the resting potential is MOSTLY being influenced by K⁺. And this matches what we know about channels -- in cells at rest, the K⁺ channels are generally open and others are generally closed.

Simplifying assumptions

In mathematics, the conditions above are known as "simplifying assumptions". There's a reason for making these assumptions -- they make the equations a lot easier to deal with.

The Nernst equation has more simplifying assumptions than the longer Goldman equation. You may have noticed we never actually used the Goldman equation to calculate anything -- in part that is because you need a lot more information in order to use the Goldman equation, including concentrations of all the ions and their permeabilities in metres per second!

The Nernst equation only requires 2 pieces of information -- the concentration inside and outside the cell. But, it comes up with a rather idealistic answer that doesn't (exactly) fit reality.