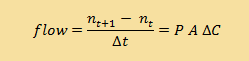Table of Contents

• Prequiz...
• Developing the Equations
• 1: Review of diffusion equations
• 2: The two-compartment model
• 3: What does continuous mean?
• 4: Examples of permeability
• 5: A formula for permeability
• 6: The area of the membrane
• 7: The final equations
• Examples
• 8: Rate of flux
• Discrete vs. Continuous
• 9: A metaphor
• 10: Continuous curves
• 11: Discrete curves
• 12: Iterating the discrete curve
• Review
• 13: Summary

Iterating the discrete curve

We compared the shape of the curves given by the two models. Solving the continuous model here is beyond the scope of this module (that would get into a lot of calculus), but we can do the discrete model with a modest amount of algebra.

Let's go back to sugar water, with the following assumptions:

• Initial concentration on left = 1 M
• Initial concentration on right = 0 M
• P = 0.1 cm/sec
• A = 1 cm²
• equal volumes on both sides of membrane

We want to know what the final concentrations will be over time , i.e., at time 1 sec, 2 sec, 3 sec, 4 sec....... So let's set up our equation from above. We know that :

Δn/Δt = 0.1 cm/sec x 1 cm² x (1 M − 0 M) = 0.1 moles/sec

In the first second, approximately 0.1 moles change sides (from the more concentrated to the less concentrated side). That leaves

on the left side: 1 mole - 0.1 moles= 0.9 moles/litre

on the right side: 0 moles + 0.1 moles= 0.1 moles/litre

How about the concentrations at time 2 seconds? We need to readjust our estimate of diffusion rate by plugging in the new concentrations. Everything else stays the same:

Δn/Δt = 0.1 cm/sec x 1 cm² x (.9 M - .1 M) = .08 moles/sec

and the new concentrations are 0.82 M and 0.18 M.

on the left side: 0.9 moles - 0.08 moles= 0.82 moles/litre

on the right side: 0.1 moles + 0.08 moles= 0.18 moles/litre

Continuing on like this,

Δn/Δt t at 3 seconds = 0.074 moles/sec,
C on left = 0.746 M, C on right = 0.254 M

Δn/Δt t at 4 seconds = 0.049 moles/sec,
C on left = 0.697 M, C on right = 0.303 M

Δn/Δt t at 5 seconds = 0.039 moles/sec,
C on left = 0.658 M, C on right = 0.342 M

Δn/Δt t at 6 seconds = 0.032 moles/sec,
C on left = 0.626 M, C on right = 0.374 M

This process is called "iteration" -- you iterate, or repeat, the equation over and over, each time substituting in the values calculated in the last iteration. You can see that, if we continued iterating long enough, we would eventually reach a point where the two concentrations were equal -- in other words, an equilibrium. (It would take a long time though, better use a spreadsheet rather than a calculator!) If you graph the two sets of concentrations, you'll see something like this: