Table of Contents

• Testing differences
• 1: How to convince your boss
• 2: Looking at the data
• 3: Is the average different?
• 4: How big is big?
• 5: How to combine SDs
• 6: Combining SDs for fun and profit
• t-calc and t-crit
• 7: Getting to t-calc
• 8: A formula for t-calc
• 9: Degrees of freedom
• 10: The bottom line (finally)
• Putting it all together
• 11: Give it a try
• 12: Once more for luck
• 13: The big picture

Let's look at the data

Luckily there is a statistical test that is perfect for your situation, and (big surprise here) it is called a t-test.

You use a t-test to find out if the averages of two sets of data are the same or not.

The first thing to get out of the way is that statisticians obviously have a different meaning for the word "same" than the rest of us. "Same" for a statistician means something like "close enough that any differences are due to chance."

For example, let's say you fed an identical diet to two identical tanks of fish. After a few weeks you found that some fish gained a few extra grams and some didn't, so overall the average weight gain was 299 grams in the first tank and 300 grams in the second tank. A statistician could probably show that the weight gains were the "same" in both tanks, even though a first-grader can tell you that the numbers "299" and "300" are not "the same".

Statisticians even have a word for this (three words, actually): "not statistically significant". If a difference is "not statistically significant", that means "the averages are so close that any differences are probably just due to chance". 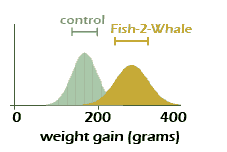On the other hand, what you (the bright young fishfood innovator) are trying to prove is that the differences between fish that eat or don't eat Fish-2-Whale ARE statistically significant.

So, getting back to our fish data, we can look at the graph and see that the distributions do not seem to overlap much, and the averages look like they are far apart. The t-test will (we hope) allow us to confirm this visual impression. It is important to realize that the t-test has no super-powers -- if your data doesn't contain a real difference, a t-test will not magically make one for you. Look at the data below:

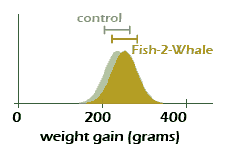
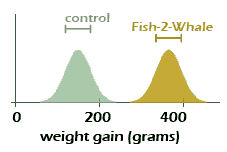
will NOT be stat. sig.	"gray zone": might be stat. sig.	will be stat. sig.

 

The take home message? If your data has an obvious difference or lack of difference, that will simply be confirmed by a t-test. BUT... if your data falls in a "gray zone" where it's hard to tell, that's when the t-test will actually help you make a decision.