MathBench > Population Dynamics

Mystery of the Missing Housefly

...mathematically expressed

Since this is a math module, you know there will be an equation in here somewhere... Let's start by calling the carrying capacity "K" (ecologists are not known for winning spelling bees). We can think of the carrying capacity (K) as the number of 'slots' available for the population. The reasoning on the last screen was, if there are a lot of 'slots' left, then the growth rate will be high.

But how many slots is 'a lot'? If K=100, then 50 slots might be a lot -- but if K=1 million, 50 slots is not much in comparison. What we really need to know is how the number available compares to the carrying capacity.

Let's try an example: If K=100 and there are 95 flies (N=95), then there are 5 slots left, right? So the number of slots left is (K-N). We could turn this into the percentage of slots left if we divide by the maximum number of slots, like this: (K-N)/K, in other words, in our example, there are 5 out of 100 = 5/100 = 5% of slots left.

Once again,

% slots left = (K-N)/K

And here's a concrete example:

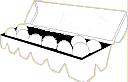
The capacity of an egg carton to carry eggs is 12 -- there are 12 slots for eggs.

In this egg carton, there are only (presently) 9 eggs. Therefore there are 12-9=3 slots available.

The proportion of slots available is 3 out of 12, or 3/12, or 25%.

Make sure you understand how this works, as it is the key to understanding the equation on the next page.

If the carrying capacity in a room is 250 flies, and there are currently 100 flies buzzing around, what percentage of the 'slots' are available?

(To make this problem interactive, turn on javascript!)

I think I have the answer: 60%