What's special about Km?
So far, we know what Vmax represents, but we still don't know how to figure out what it is without blowing the budget. On the other hand, we don't even know what Km represents, much less how to find the value experimentally! Think about this:
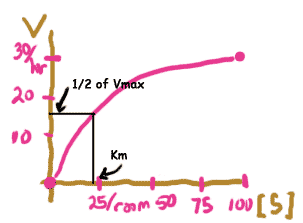
at what [S] do you expect to get to exactly 1/2 of Vmax?
(To make this problem interactive, turn on javascript!)
- I need a hint ... : you need the chunk [S]/(Km + [S]) = 0.5
- ...another hint ... :Km + [S] must be twice as big as [S]
I think I have the answer: Km = [S]
It turns out that Km is exactly the concentration of substrate for which the reaction runs at half its maximum rate. In chocolate terms, Km is exactly the number of bags of chocolate chips per square meter needed to achieve exactly half of the maximum goop production rate.
Coincidence? Nope.


| If you turn on javascript, this becomes a rollover |
So, according to this graph, the maximum rate of goop production is 30 bags per hour, and if I put out 24 bags / room (=Km), then I get half the maximum rate, or 15 bags per hour converted to goop. Thus, the equation that produced this graph must be:

Copyright University of Maryland, 2007
You may link to this site for educational purposes.
Please do not copy without permission
requests/questions/feedback email: mathbench@umd.edu