Table of Contents

• Prequiz...
• 1: The Case of the Confusing Axes
• 2: Choose your x and y carefully
• 3: Practise labelling the axes
• 4: Next step: ranging the axes
• 5: Practise ranging the axes
• 6: Next step: scale the axes
• 7: Practise determining labelled interval distance
• 8: How to scale axes
• 9: Finally, fill in the axes
• 10: Summary

Learning Outcomes

After completing this module you should be able to:

• Create a graph by hand.
• Present data in a graph.

The case of the confusing axes

In this module we'll talk about how to graph data, and particularly how to set up the graph.

Below you will find an easy checklist for making the perfect graph. On the following pages, we'll practise some of the skills you will need...

Checklist:
• Choose your x and y variables carefully.
• Label your x and y axes using both a description and the units in parentheses (brackets).
• Label the top of the graph with a description of what the graph shows.
• Figure out the best minimums and maximums for x and y.
• Figure out the best distance for tick marks or intervals on each axis.
• Include a legend if there's more than one data series.
• Graph the data!

I'm going to quickly show you these steps on a single graph, and then as we go through each step more slowly, I'll show you the sorts of trainwrecks that occur if you ignore these steps.

A sample graph: eyesight and TV viewing distance

When I was growing up, the older folks frequently rained dire predictions on our heads about our TV viewing habits. Specifically, what happens to our eyesight if we were to sit too close to the TV. Anyway, I could imagine my poor grandmother collecting the data for the graph below, hoping to prove her point (that sitting too close to the TV results in poor eye sight) and forcing us to sit further from the TV screen:

viewing distance	10	20	30	40	50	60	70	80	100	120	140
eyesight remaining	75	80	85	88	92	95	97	98	98	98	98

 

• Choose the x and y variables:
  x: viewing distance is the independent variable
  y: eyesight remaining is the dependent variable (because it depends on viewing distance)
• Label your x and y axes:
  x: "distance from TV (cm)"
  y: "eyesight left (%)"
  graph heading: "Effect of TV viewing distance on eyesight"
• Minimums and maximums
  x: 0 to 150
  y: 0 to 100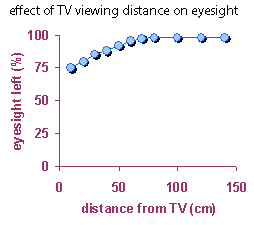
• Tick mark (interval) distance
  x: 50
  y: 25
• Legend (if there's more than one data series)
  Lucky us, we don't need a legend
• Graph the data!